Street lighting is an essential part of urban infrastructure, providing safety and visibility during the night. However, the structural integrity of the lighting columns can sometimes be compromised due to various stresses.
One solution is to cut back the street lighting bracket, effectively raising the height of the luminaire from the ground. But does this adjustment necessitate an increase in energy or power to maintain the same level of illuminance? Let’s delve into the science behind it.
The Science of Illuminance
Illuminance (E) is a measure of how much luminous flux is spread over a given area and is given by the formula:
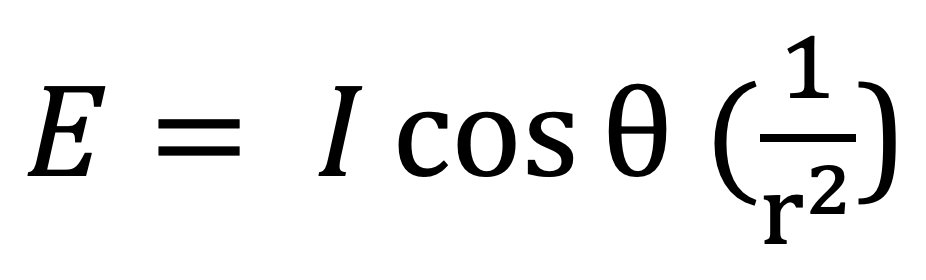
Where I is the luminous flux, cosθ is the cosine of the angle of incidence, and r is the distance from the light source to the ground.
The Inverse Square Law
The term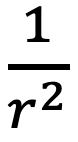 
in the equation signifies that illuminance decreases with the square of the distance from the light source. This is known as the inverse square law. If you double the distance (r), the illuminance (E) will be reduced to a quarter of its original value.
Energy Consumption and Illuminance
The energy consumed by a light source is often given by the formula P=IV, where P is the electrical power in watts, I is the current in amperes, and V is the voltage in volts. To relate this to illuminance, we consider the luminous efficacy (η) of the light source:
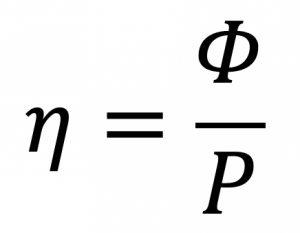
which can be rearranged to
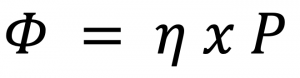
Substituting this into the original illuminance equation, we get:
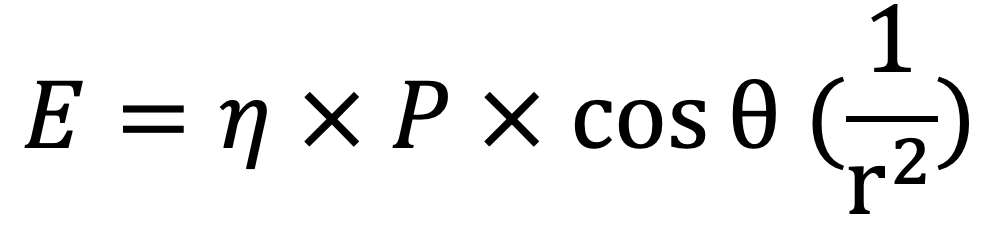
The Impact of Changing Distance
If the distance (r) from the luminaire to the ground increases, say from 10m to 11m, and you wish to maintain the same illuminance (E), then we can set up the following equation (noting that cosq is likely to alter but for simplicity I am not adjusting this):
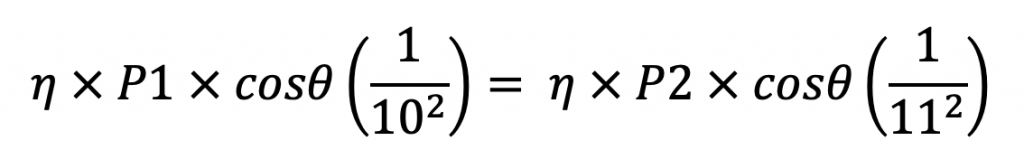
Since η and cosθ are constant, they can be cancelled out:
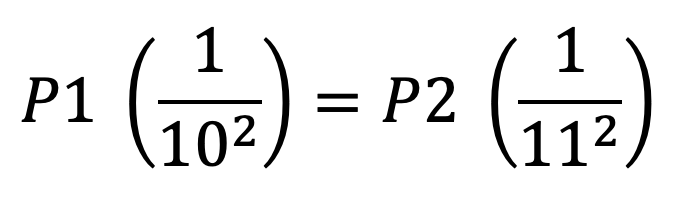
Solving for P2, the power needed to maintain the same illuminance E when r changes from 10m to 11m, we get:
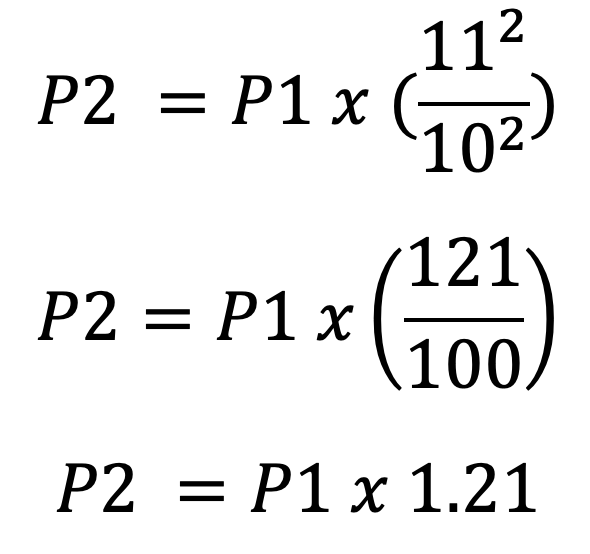
So, the power needed at 11m would be 1.21 times the original power at 10m to maintain the same illuminance E. This would likely result in an increase in energy consumption of 21%.
But part of the reason for cutting this street lighting bracket back is likely to be to install a more energy efficient luminaire – do I still achieve an energy saving even when I cut the bracket back?
A Practical Example
Let’s say the original power was 50W at a height of 10m. If you cut back the bracket to increase the luminaire distance to the road to 11m, but reduce the luminaire power to 15W, for a nice new LED, the percentage change in power needs would be:
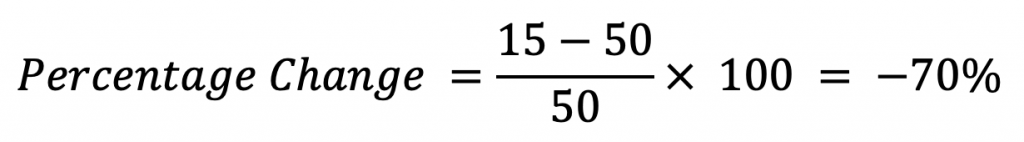
However, to maintain the same illuminance, the new power would need to be
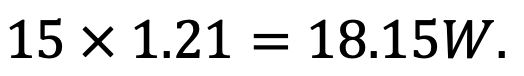
Even after adjusting the power to 18.15W to maintain the same illuminance at the new height, we still achieve a significant power saving compared to the original 50W. The new percentage change in power needs, when compared to the original 50W, would be:
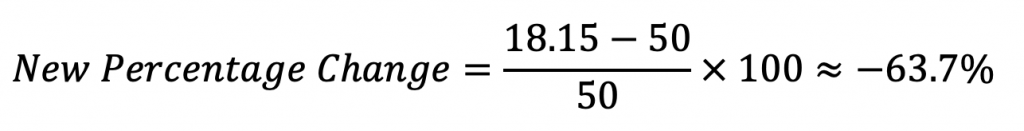
This means that even with the adjustment to maintain illuminance, a power saving of approximately 63.7% is still achieved (originally 70%). Therefore, cutting back the bracket not only helps in reducing stresses on the column but also allows for considerable energy savings, even when adjustments are made to maintain the same level of illuminance.
The Structural Benefits of Cutting Back the Bracket
When considering the adjustment of street lighting infrastructure, it’s crucial to understand the structural implications. Cutting back the street lighting bracket offers several advantages in terms of reducing various stresses on the column. Here’s how:
Reduced Bending Stress: Shortening the bracket effectively lessens the bending moment on the column. This is particularly beneficial at the base, where bending moments are usually at their peak.
Mitigated Torsional Stress: If the luminaire is not aligned with the column’s centreline, a shorter bracket will decrease the torsional stresses, enhancing the column’s stability.
Lower Wind Loading: A more compact bracket exposes less surface area to wind forces, thereby reducing the wind-induced moments and stresses on the column.
Decreased Vibrational Stress: A shorter bracket is generally less prone to vibrations from external factors like wind or passing traffic. This minimises fatigue stresses that can accumulate over time.
Minimal Shear Stress: While the impact on shear stress is less significant compared to bending and torsional stresses, a shorter bracket may still offer slight reductions in shear forces.
Lighter Dead Load: The weight of the luminaire and the bracket contribute to the overall load on the column. A shorter, and potentially lighter, bracket can reduce this dead load, further enhancing structural integrity.
Conclusion
Cutting back the bracket on a street lighting column offers a range of structural benefits, from reducing various types of stresses to enhancing overall stability. This adjustment also presents an excellent opportunity to upgrade to more energy-efficient lighting solutions, such as making the switch from traditional SON lamps to modern LED technology.
While raising the luminaire increases the distance to the ground, which could theoretically require a slight uptick in power to maintain the same level of brightness, the energy savings from using LED technology remain significant. In essence, even if you need to adjust the power slightly to keep the same level of illuminance, the overall energy consumption is still markedly reduced when transitioning to LEDs.
In summary, cutting back the bracket not only improves the structural integrity of your lighting column but also allows for considerable energy savings. The end result is a street lighting system that is both safer and more energy-efficient, without compromising on brightness.